Trigonometric Functions

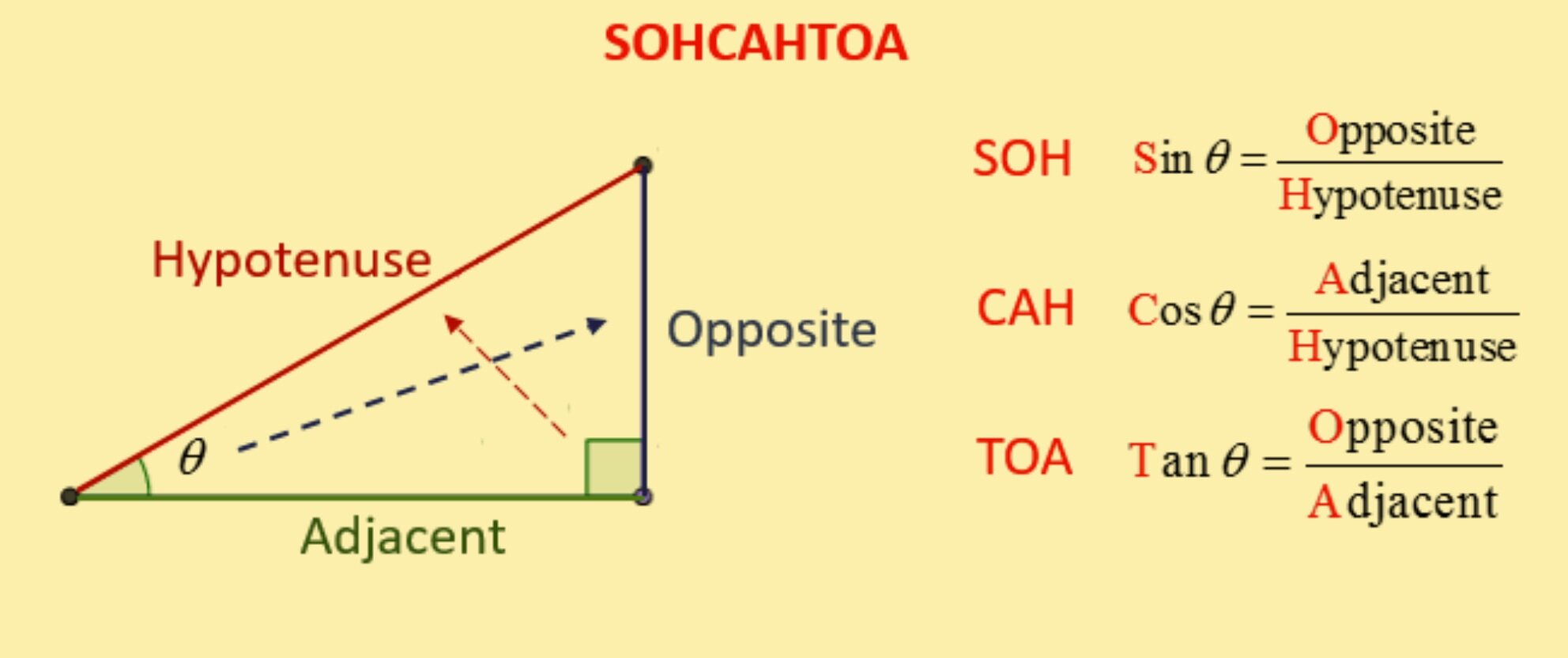
The foremost and probably the most important functions of trigonometry are sine, cosine, and tangent. Using these, you can solve for unknown angles and side lengths in any right-angled triangle! Right-angled triangles are differentiated from other triangles in that they all have one 90° angle. To solve for the sine of an angle, you would use the equation sin(θ)=opposite/hypotenuse. The “hypotenuse” is always the length of the longest side of a right triangle, and the “opposite” is always the length of the side opposite to the angle being solved for or the angle given. Next, to solve for the cosine of an angle and the tangent of an angle, you would correspondingly use the equations cos(θ)=adjacent/hypotenuse and tan(θ)=opposite/adjacent. The “adjacent” is the length of the side that is next to the angle being solved for or the angle given.

As long as you know two lengths of a right triangle, you can solve for an unknown angle. Likewise, as long as you know an angle and a side of the triangle, you can solve for an unknown side in the triangle. To illustrate, imagine a right triangle with a side the length of 3 units, a side the length of 4 units, and a side the length of 5 units. To find the measure of the angle opposite of the side with the length of three, use the equation sin(θ)=opposite/hypotenuse. Worked out, the problem looks like this—
sin(θ)=opposite/hypotenuse
sin(θ)=3/5
θ=sin^-1(3/5)
θ≈36.87

In the third step, theta, or the Greek letter θ used in mathematics to represent an angle, is isolated on one side of the equation by taking the inverse sine of both sides. Finally, in the last step a calculator is used to find the inverse sine of 3/5 and the outcome is approximated to the nearest hundredth. With enough given variables, any right triangle can be solved with sine, cosine, and tangent, and the idea expands beyond that of right triangles! Understanding of these trigonometric functions can help you learn further impressive concepts such as other trigonometric identities and the unit circle. In mathematics, no solution is too far with the right (pun) equations!

Picture Source: onlinemathlearning.com