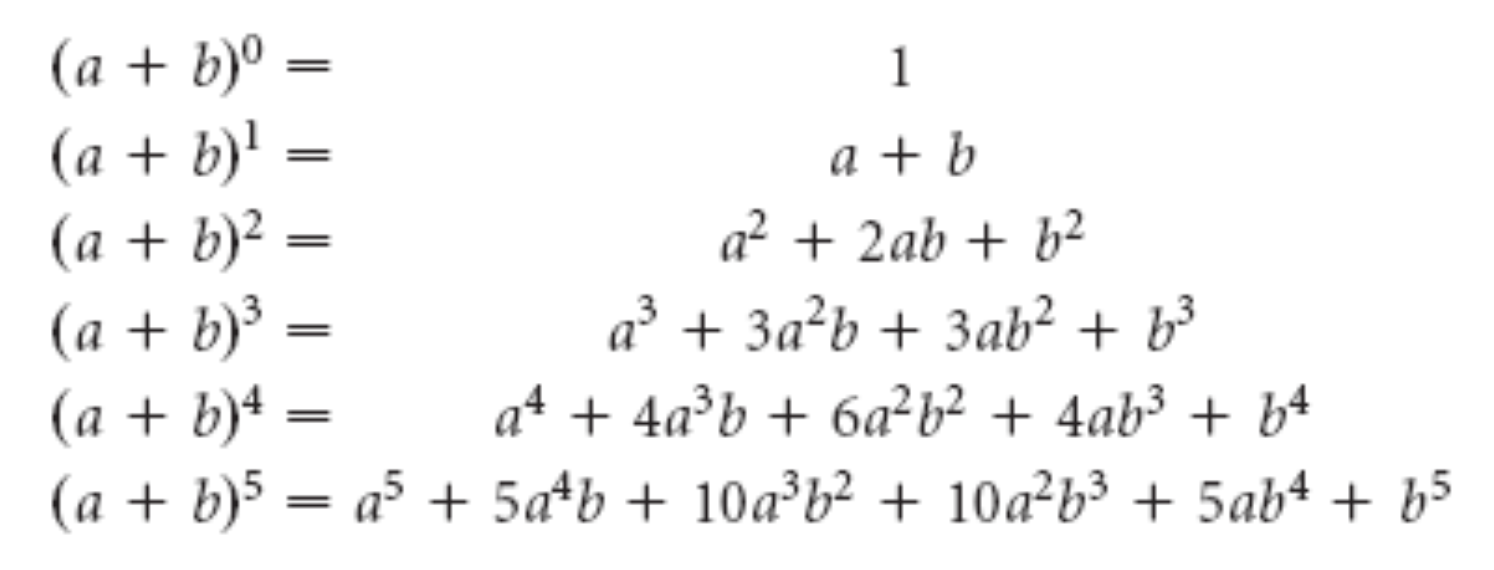In areas such as calculus and algebra, you will often be asked to expand binomials. Binomials are basically algebraic expressions that include two terms. For example, (a+b) is a binomial. Any numbers can be substituted for the terms a and b. If a is substituted with 2 and b is substituted with 3, (a+b)=(2+3)=5. Simple, right! Binomial expansion is the act of expanding the expression (a+b)^n. In binomial expansion, one can easily use the FOIL method, which stands for Forward, Outer, Inner, and Last. It’s a fun technique that goes on to be used even in upper math courses. Here’s an example problem for review of this technique.

Here, we are going to expand the expression (a+b)^2 in three steps.
(a+b)^2=(a+b)(a+b)
=a^2+ab+ba+b^2
=a^2+2ab+b^2
In the first step, (a+b)^2 is rewritten as (a+b)(a+b). In the second step, the FOIL method is used, multiplying a times a (Forward terms), a times b (Outer terms), b times a (Inner terms), and b times b (Last terms). In the third step, the result is simplified by combining like terms.

However, how do you approach a problem where you are asked to solve (a+b) raised to the power of a high number, such as seven? That’s a lot of FOILing! Luckily, this is where Pascal’s Triangle comes into play (refer to the chart at the bottom of this article). Pascal’s triangle allows us to visualize the outcome by giving us the values of the binomial coefficients. Don’t worry if this doesn’t make sense to you right now. It will become clearer so be patient! Pascal’s Triangle is often simplified for memorization purposes (as shown in the picture), but if written in depth it would look like this.

The numbers in Pascal’s Triangle represent the coefficients of each term in the outcome. The exponents of a and b are then given by a pattern. The first variable of the term, or a in this case, is introduced in the first term and is always raised to the power of whatever number the original algebraic expression is being raised to. The second term of the binomial, or b, is then introduced in the second term and is always raised to the power of 1. At the same time, in the second term, the exponent of a lowers in value by 1. With each term, the exponents of a will continue to lower until the it reaches 1 as the exponents of b will continue to rise until it matches the exponent of the original algebraic expression. Using this pattern and the binomial coefficients given to us in Pascal’s Triangle helps students and professional mathematicians expand binomials easily!